Sunday, April 08, 2018
Thursday, September 25, 2008
Math Practice Problems #2
Today, I was asked this question:
"Titan and Rhea are the two largest moons of Saturn. The ratio of of the surface area of Titan to the surface area of Rhea is about 34:3. If the diameter of Rhea is about 1530km, what is the diameter of Titan?"
This one's pretty simple. We only need two formulae.
A=4πr2
d=2r
d=2r
That's for the surface area of a sphere. Now we need to assign values.
ATitan=4πrTitan2
ARhea=4πrRhea2
dRhea=1530 km
ATitan=(34/3)ARhea
ARhea=4πrRhea2
dRhea=1530 km
ATitan=(34/3)ARhea
Now we can start figuring out the diameter of Titan
rRhea=1530km/2=765km
4πrTitan2=(34/3)4π(7652)
4πrTitan2=(34/3)4π(7652)
Since there's a 4π on each side, we can eliminate it from both sides.
rTitan2=(34/3)(7652)
Next, we take the square root of both sides
rTitan=√((34/3)(7652))
Now we multiply both sides by 2.
dTitan=2·√((34/3)(7652))
↓
dTitan=5150.74752
↓
dTitan=5150.74752
Finally, we need to round the answer until the number of digits we end with matches the number we started with, so the final answer is 5150 km.
Wednesday, September 24, 2008
Physics Practice Problems #1
OK, so someone asked for help with this problem.
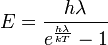
Heating 235g of water from 22.6C to 94.4C in a microwave oven requires 7.06 x 104J of energy. If the microwave frequency is 2.88 x 105s-1, how many quanta are required to supply the 7.06 x 104J?
You only really need from the question are the frequency of the microwaves (2.88•105s-1), the temperature change (22.6C to 94.4C), and the total energy (7.06•104J). First, we turn the frequency into a wavelength.
λ=c/f
c=299,792,458 m/s
f=2.88•105s-1
λ=299,792,458/(2.88•105) m•s/s
λ=1040.49603
c=299,792,458 m/s
f=2.88•105s-1
λ=299,792,458/(2.88•105) m•s/s
λ=1040.49603
Next, we need to find the temperature change.
T0=22.6°C=295.75K
T1=94.4°C=367.55K
T0=22.6°C=295.75K
T1=94.4°C=367.55K
T=T1-T0
T=367.55-295.75
T=71.8
T=367.55-295.75
T=71.8
Next, we'll need a few constants.
Planck's Constant: h=6.626068•10-34 m2kg/s
Boltzmann's Constant: 1.3806503•10-23 m2•kg•s-2•K-1
e=2.71828183
Now, we're ready to find the energy of one photon, but we need a formula.
Planck's Constant: h=6.626068•10-34 m2kg/s
Boltzmann's Constant: 1.3806503•10-23 m2•kg•s-2•K-1
e=2.71828183
Now, we're ready to find the energy of one photon, but we need a formula.
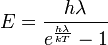
You use all the values in that formula and you get 9.91306903•10-22 J per photon.
Now all you need to do is divide the total energy by the amount of energy each photon caries.
Now all you need to do is divide the total energy by the amount of energy each photon caries.
(7.06•104J)/(9.91306903•10-22J)
7.12191147•1025
7.12191147•1025
So it takes 7.12191147•1025 photons to heat the water, but we need to remember to round to the appropriate number of digits. We started wit 3-digit numbers, so we have to end with a 3-digit number. The final answer is 7.12•1025 photons.
Thanks to Wikipedia for the picture of the formula.
Thanks to Wikipedia for the picture of the formula.
Energy
There are different forms of energy, like electrical, thermal and chemical, but I'd like to start off with mechanical energy.
Well, what is energy? Energy is the amount of work that gets done. It is the amount of force applied to something for a given distance. There was this saying in my high school physics class: "Work is mad". That means that the amount of work you do (energy you use) on an object is equal to the mass, acceleration and distance multiplied together. I'll give you two formulas right now.
Well, what is energy? Energy is the amount of work that gets done. It is the amount of force applied to something for a given distance. There was this saying in my high school physics class: "Work is mad". That means that the amount of work you do (energy you use) on an object is equal to the mass, acceleration and distance multiplied together. I'll give you two formulas right now.
E=mad
E=Fd
E=Fd
The unit for energy is called the joule. It's abbreviated as J and can be written in terms of the unit's we've already learned about.
1J=1Nm
1J=1 m2kg/s2
1J=1 m2kg/s2
OK, here's a problem: We have a crate that weighs 500 Newton that needs to be lifted 39 meters to a 13th-story window. How much energy will it take to lift straight up?
F=500kg
d=39m
F=500kg
d=39m
E=Fd
E=500N·39m
E=19500 N·m
E=19,500 J
E=500N·39m
E=19500 N·m
E=19,500 J
So you'd need to use 19,500 joules of energy to get the crate up to the window.
Tuesday, September 02, 2008
Math Practice Problems #1
While out roaming the Internet, I sometimes encounter people who need help with their homework, so I had the idea of collecting their questions and posting them here.
"If the polynomial 4-7x+2x2-5x3 has degree a, leading coefficient b, and constant term c, then the value of 3a-2b-c is _____."
"If the polynomial 4-7x+2x2-5x3 has degree a, leading coefficient b, and constant term c, then the value of 3a-2b-c is _____."
Well, the highest exponent is 3 from -5x3,and the leading coefficient is always attacher to the highest exponent, so that's -5. The constant is the term that doesn't have an exponent, so that's 4.
So...
a=3
b=-5
c=4
And we can solve the problem.
So...
a=3
b=-5
c=4
And we can solve the problem.
3a-2b-c
3(3)-2(-5)-4
9-(-10)-4
9+10-4
15
3(3)-2(-5)-4
9-(-10)-4
9+10-4
15
And the answer is 15.
The Periodic Table
There are 118 known elements in the world. Some happen naturally and some are made in a lab. With all those elements, it's easy to get lost if you don't have a good system for sorting them. Fortunately, chemists and the alchemists before them thought of this problem. As they learned more about different elements, they worked out a pretty good way of sorting them called the Periodic Table of Elements.

You can get some very important information just by looking at the Periodic Table, like how many valence electrons it has, how hard it is to make 2 atoms bond, or if they'll even bond at all.

You can get some very important information just by looking at the Periodic Table, like how many valence electrons it has, how hard it is to make 2 atoms bond, or if they'll even bond at all.
Monday, September 01, 2008
Tangent
Hello, everyone, I'm back from my year-long absence. I promised that I'd write about the tangent (tan) that went with sine and cosine in trigonometry, so here goes. First, we take the same triangle we used for the sine and cosine.

Let's use the same axes, too.
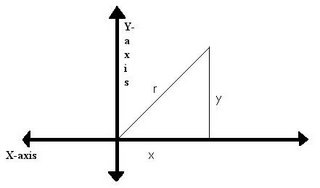
On the first triangle, A, B, and C still represent the sizes of the angles in degrees. The little a, b, and c represent the lengths of each side. Here's how they combine to form tangential measurements:
Remember the "Toa" part of "SohCahToa"? It stands for tangent equals opposite over adjacent. On the axis drawing, x is adjacent and y is opposite, so

Let's use the same axes, too.

On the first triangle, A, B, and C still represent the sizes of the angles in degrees. The little a, b, and c represent the lengths of each side. Here's how they combine to form tangential measurements:
tanA=a/c
tanC=c/a
tanC=c/a
Remember the "Toa" part of "SohCahToa"? It stands for tangent equals opposite over adjacent. On the axis drawing, x is adjacent and y is opposite, so
tanθ=y/x
Wednesday, May 02, 2007
Force #2
Now, gravitational force, normal force, what are they? What do they have to do with each other?
Well here it is. Gravitational force is the force pulling something down. Normal force… You know how when there’s something on a table, the thing doesn’t sink into the table, but the table doesn’t launch it either? Normal force does that. Normal force is the force an object exerts to counter the force of another object trying to push through it. It’s usually gravity’s equal and opposite reaction. I’ll show you what I mean.
Let’s show up at a beach in southern India, that’s close enough to sea level and the Equator. Let’s bring a 2500 kilogram elephant with us while we’re at it.
Well here it is. Gravitational force is the force pulling something down. Normal force… You know how when there’s something on a table, the thing doesn’t sink into the table, but the table doesn’t launch it either? Normal force does that. Normal force is the force an object exerts to counter the force of another object trying to push through it. It’s usually gravity’s equal and opposite reaction. I’ll show you what I mean.
Let’s show up at a beach in southern India, that’s close enough to sea level and the Equator. Let’s bring a 2500 kilogram elephant with us while we’re at it.
2500Kg•9.81m/s2
↓
2500•9.81=24525
↓
24525N
↓
2500•9.81=24525
↓
24525N
The elephant weighs 24525 Newtons. That being true, it exerts 24525 Newtons of force on the ground just by standing there, and that force acts in a downward direction. The elephant doesn’t sink into the ground, nor does it get launched up into the air, and is pretty happy with that. So, what conclusion can we gather from that? The normal force is equal to the elephant’s weight, but in the opposite direction, pushing up against the elephant’s feet. Yes, gravitational force is 24525N down, so normal force is 24525N up.
Subscribe to:
Posts (Atom)